Read on Twitter
Finally finished my piece on flexible NHL aging. I'll post a couple of important things in a thread here, but before any of that, here is the link to the piece. http://rpubs.com/cjtdevil/nhl_aging
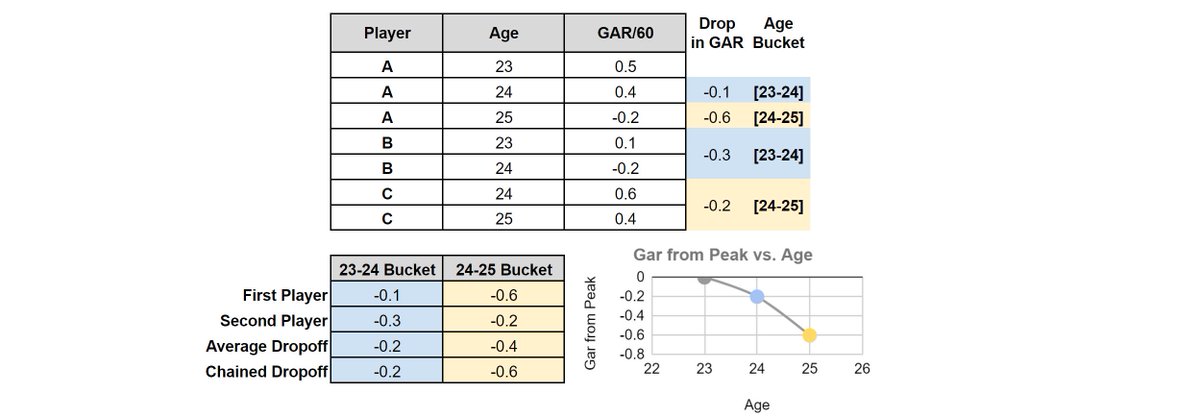
The piece is in 3 parts. In part 1, I talk about the basics of aging including the standard delta method, why I prefer regression, and a commentary on the impact of "survivor bias"
There is very little math in this part, and is pretty generalizable.
There is very little math in this part, and is pretty generalizable.
In part 2, I explain the logic behind GAMs in this context, and show some preliminary results on what aging curves would look like if built off that foundational model --
gam( gar60 ~ player + s(age) )
This is also not TOO technical.
gam( gar60 ~ player + s(age) )
This is also not TOO technical.
In part 3, I explain a potential method -- tensor-products -- for making aging curves flexible without losing the generalizability of the model:
gam( gar60 ~ player + te(age, decay_rate) )
This one is a little more technical, but also produces pretty pictures like this.
gam( gar60 ~ player + te(age, decay_rate) )
This one is a little more technical, but also produces pretty pictures like this.
Thanks to @EvolvingWild, @jc_bradbury, @tangotiger, @IneffectiveMath, @903124S, and @garik16 all of whom I spoke with about this piece at some point, as well as @RK_Stimp, @iyer_prashanth, and @pflynnhockey who helped me edit.
I worked on this for about 2 months and I don't think it's perfect, but I've been procrastinating for too long, so if you can make it through it, let me know what you think -- good or bad.
